

The symbol * is used in spreadsheets and other computer applications to indicate a multiplication, although * does have other more complex meanings in mathematics. These symbols have the same meaning commonly × is used to mean multiplication when handwritten or used on a calculator 2 × 2, for example.
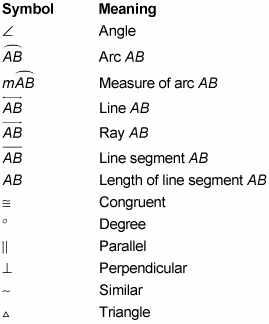
#GEOMETRY SYMBOLS DEFINITIONS PLUS#
Our page on Positive and Negative Numbers explains that a number without a sign is considered to be positive, so the plus is not usually necessary. The + symbol can also be used to indicate a positive number although this is less common, for example, +2. The addition symbol + is usually used to indicate that two or more numbers should be added together, for example, 2 + 2. This page complements our numeracy skills pages and provides a quick glossary of common mathematical symbols and terminology with concise definitions.Īre we missing something? Get it touch to let us know.Ĭommon Mathematical Symbols + Addition, Plus, Positive Mathematical symbols and terminology can be confusing and can be a barrier to learning and understanding basic numeracy. Understanding Statistical Distributions.Area, Surface Area and Volume Reference Sheet.Simple Transformations of 2-Dimensional Shapes.Polar, Cylindrical and Spherical Coordinates.Introduction to Cartesian Coordinate Systems.Introduction to Geometry: Points, Lines and Planes.Percentage Change | Increase and Decrease.Mental Arithmetic – Basic Mental Maths Hacks.Ordering Mathematical Operations - BODMAS.Common Mathematical Symbols and Terminology.Special Numbers and Mathematical Concepts.How Good Are Your Numeracy Skills? Numeracy Quiz.Basically, you look at the sheaf of differential operators on your variety, construct a degree filtration of that sheaf, then the corresponding graded sheaf is isomorphic to the direct image of the sheaf of regular functions on the cotangent bundle via the symbol map. The coordinate-free generalization for say smooth quasi-projective varieties over the complex numbers is done in Chapter 2, section 3. Of course, this section only covers the (complex) affine case you already describe. This goes through the construction of the filtration Ben mentions, then constructs the graded module and symbol map explicitly. The D-module course notes of Dragan Milicic contain a detailed construction of the symbol map - they can be found on his webpage There may be several versions linked there - the 2007-2008 course should be most thorough. This gives a way to define the principal symbol on manifolds, which of course agrees with the standard definition.


One can construct wave packets in arbitrary smooth manifolds, basically because they look flat at small scales, and one can define the inner product $\xi_0\cdot(x-x_0)$ invariantly (up to lower order corrections) in the asymptotic limit when $x$ is close to $x_0$ and $(x_0,\xi_0)$ is in the cotangent bundle. (This is why one has a pseudodifferential calculus.) The diagonal coefficients are essentially the principal symbol of the operator. (The lower order terms are related to the lower order components of the symbol, but the precise relationship is icky.)īasically, when viewed in a wave packet basis, (pseudo)differential operators are diagonal to top order. This number $a(x_0,\xi_0)$ is the principal symbol of $a$ at $(x_0,\xi_0)$. When one does so (using the chain rule and product rule as appropriate), one obtains a bunch of terms with different powers of $1/\hbar$ attached to them, with the top order term being $1/\hbar^d$ times some quantity $a(x_0,\xi_0)$ times the original wave packet. Now apply a differential operator $L$ of degree $d$ to this wave packet. I think I understand the basic idea on $\mathbb$ for some smooth cutoff $\eta$ and some small $\epsilon$ (but not as small as $\hbar$). I find Wikipedia's discussion of symbols of differential operators a bit impenetrable, and Google doesn't seem to turn up useful links, so I'm hoping someone can point me to a more pedantic discussion.


 0 kommentar(er)
0 kommentar(er)
